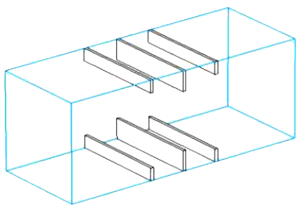
Analytical and numerical method of constructive synthesis of optimal polarizers based on three irises in square waveguide
DOI:
https://doi.org/10.3103/S073527272104004XKeywords:
waveguide polarizer, square waveguide, iris, equivalent circuit, iris inductive susceptance, iris capacitive susceptance, scattering matrix, transmission matrix, differential phase shift, voltage standing-wave ratio, VSWR, axial ratio, cross-polarization decoupling, CPDAbstract
This paper proposes an approximate analytical and numerical constructive synthesis method of optimal waveguide polarizers based on three irises in square waveguide. A mathematical model of polarizer has been developed on the basis of waveguide transmission and scattering matrices. The analytical expressions derived for elements of the scattering matrix were used for determining the electrical characteristics of polarizer. The synthesis conditions were developed in the form of a system of equations, the solution of which should ensure the minimal deviation of the differential phase shift with respect to 90°, the maximum possible flat phase frequency characteristic, and the best matching in the specified frequency band. The method was evaluated on the constructive synthesis of polarizers for three working frequency bands: 7.25–8.6, 7.75–8.5, and 8.0–8.5 GHz. The validity of constructive synthesis results was confirmed by the calculation data of characteristics of synthesized polarizers using the finite element method in frequency domain employing the CST Microwave Studio Software. A satisfactory matching of electrical characteristics calculated by both methods was achieved. For the working frequency band of up to 10%, the proposed method of constructive synthesis ensures a sufficient for practical purposes accuracy in determining the dimensions of polarizer and the corresponding electrical specifications. This method can be also recommended for rapid determination of initial values of polarizer dimensions guaranteeing the operation in the vicinity of global optimum and thereby speeding up the designing of optimal polarizers at the electrodynamic level. In addition, this method can be extended to the constructive synthesis of waveguide polarizers with a large number of irises.
References
- A. J. Simmons, “A compact broad-band microwave quarter-wave plate,” Proc. IRE, vol. 40, no. 9, pp. 1089–1090, 1952, doi: https://doi.org/10.1109/JRPROC.1952.273879.
- A. J. Simmons, “Phase shift by periodic loading of waveguide and its application to broad-band circular polarization,” IEEE Trans. Microw. Theory Tech., vol. 3, no. 6, pp. 18–21, 1955, doi: https://doi.org/10.1109/TMTT.1955.1124986.
- F. F. Dubrovka, O. M. Kuprii, “Synthesis of microwave phase shifters based on reactive elements in waveguide,” Radioelectron. Commun. Syst., vol. 25, no. 8, pp. 32–36, 1982.
- F. Arndt, W. Tucholke, T. Wriedt, “Design of a wideband compact square waveguide polariser,” Electron. Lett., vol. 21, no. 12, pp. 517–518, 1985, doi: https://doi.org/10.1049/el:19850365.
- R. Gruner, “Design procedure for a distributed reactance waveguide polarizer,” in 1985 Antennas and Propagation Society International Symposium, 1985, vol. 23, pp. 635–638, doi: https://doi.org/10.1109/APS.1985.1149405.
- U. Tucholke, F. Arndt, T. Wriedt, “Field theory design of square waveguide iris polarizers,” IEEE Trans. Microw. Theory Tech., vol. 34, no. 1, pp. 156–160, 1986, doi: https://doi.org/10.1109/TMTT.1986.1133293.
- B. M. Park, P. Ramunujam, F. Boldissar, C. Shin, “Sensitivity analysis of square waveguide iris polarizers,” in Proceedings of IEEE Antennas and Propagation Society International Symposium and URSI National Radio Science Meeting, 1994, vol. 2, pp. 1058–1061, doi: https://doi.org/10.1109/APS.1994.407908.
- F. F. Dubrovka, P. Y. Stepanenko, “Broadband sections of differential phase shift on a corrugated square waveguide,” Izv. Vyss. Uchebnykh Zaved. Radioelektronika, vol. 39, no. 1, pp. 3–10, 1996.
- G. Virone, R. Tascone, M. Baralis, O. A. Peverini, A. Olivieri, R. Orta, “A novel design tool for waveguide polarizers,” IEEE Trans. Microw. Theory Tech., vol. 53, no. 3, pp. 888–894, 2005, doi: https://doi.org/10.1109/TMTT.2004.842491.
- G. Virone, R. Tascone, O. A. Peverini, R. Orta, “Optimum-iris-set concept for waveguide polarizers,” IEEE Microw. Wirel. Components Lett., vol. 17, no. 3, pp. 202–204, 2007, doi: https://doi.org/10.1109/LMWC.2006.890474.
- G. Virone, R. Tascone, O. A. Peverini, G. Addamo, R. Orta, “Combined-phase-shift waveguide polarizer,” IEEE Microw. Wirel. Components Lett., vol. 18, no. 8, pp. 509–511, 2008, doi: https://doi.org/10.1109/LMWC.2008.2001005.
- A. Kirilenko, D. Kulik, Y. Parkhomenko, L. Rud, V. Tkachenko, “Automatic electromagnetic solvers based on mode-matching, transverse resonance, and S-matrix techniques,” in 14th International Conference on Microwaves, Radar and Wireless Communications. MIKON - 2002. Conference Proceedings (IEEE Cat.No.02EX562), 2002, vol. 3, pp. 815–824, doi: https://doi.org/10.1109/MIKON.2002.1017964.
- S. A. Prikolotin, S. A. Steshenko, D. Y. Kulik, L. A. Rud, A. A. Kirilenko, “Fast full 3D EM CAD of waveguide units based on the generalized mode matching technique,” in 2012 International Conference on Mathematical Methods in Electromagnetic Theory, 2012, pp. 109–112, doi: https://doi.org/10.1109/MMET.2012.6331291.
- I. Agnihotri, S. K. Sharma, “Design of a compact 3-D metal printed Ka-band waveguide polarizer,” IEEE Antennas Wirel. Propag. Lett., vol. 18, no. 12, pp. 2726–2730, 2019, doi: https://doi.org/10.1109/LAWP.2019.2950312.
- N. Zhang, Y.-L. Wang, J.-Z. Chen, B. Wu, G. Li, “Design of K/Ka-band diplex circular polarizer with high isolation,” in 2018 International Conference on Microwave and Millimeter Wave Technology (ICMMT), 2018, pp. 1–3, doi: https://doi.org/10.1109/ICMMT.2018.8563363.
- M. Zheng, J. Qi, “A low-cost high-performance dual-band diaphragm circular polarizer,” in 2020 IEEE International Symposium on Antennas and Propagation and North American Radio Science Meeting, 2020, pp. 1979–1980, doi: https://doi.org/10.1109/IEEECONF35879.2020.9329806.
- F. Arndt et al., “Fast CAD and optimization of waveguide components and aperture antennas by hybrid MM/FE/MoM/FD methods—state-of-the-art and recent advances,” IEEE Trans. Microw. Theory Tech., vol. 52, no. 1, pp. 292–305, 2004, doi: https://doi.org/10.1109/TMTT.2003.820890.
- W. Abdouni-Abdallah, M. S. Khan, A. Konstantinidis, “Design of a wideband multilayer meander-line polarizer (6 – 18 GHz) using a semi-analytical method,” in 2018 48th European Microwave Conference (EuMC), 2018, pp. 137–140, doi: https://doi.org/10.23919/EuMC.2018.8541675.
- S. I. Piltyay, A. V. Bulashenko, I. V. Demchenko, “Waveguide iris polarizers for Ku-band satellite antenna feeds,” J. Nano- Electron. Phys., vol. 12, no. 5, pp. 05024-1-05024–5, 2020, doi: https://doi.org/10.21272/jnep.12(5).05024.
- S. I. Piltyay, O. Y. Sushko, A. V. Bulashenko, I. V. Demchenko, “Compact Ku-band iris polarizers for satellite telecommunication systems,” Telecommun. Radio Eng., vol. 79, no. 19, pp. 1673–1690, 2020, doi: https://doi.org/10.1615/TelecomRadEng.v79.i19.10.
- A. V. Bulashenko, S. I. Piltyay, I. V. Demchenko, “Wave matrix technique for waveguide iris polarizers simulation. Theory,” J. Nano- Electron. Phys., vol. 12, no. 6, pp. 06026-1-06026–5, 2020, doi: https://doi.org/10.21272/jnep.12(6).06026.
- D. Blanco, R. Sauleau, “Broadband and broad-angle multilayer polarizer based on hybrid optimization algorithm for low-cost Ka-band applications,” IEEE Trans. Antennas Propag., vol. 66, no. 4, pp. 1874–1881, 2018, doi: https://doi.org/10.1109/TAP.2018.2804618.
- P. Naseri, J. R. Costa, S. A. Matos, C. A. Fernandes, S. V. Hum, “Equivalent circuit modeling to design a dual-band dual linear-to-circular polarizer surface,” IEEE Trans. Antennas Propag., vol. 68, no. 7, pp. 5730–5735, 2020, doi: https://doi.org/10.1109/TAP.2020.2963949.
- A. Bulashenko, S. Piltyay, Y. Kalinichenko, O. Bulashenko, “Mathematical modeling of iris-post sections for waveguide filters, phase shifters and polarizers,” in 2020 IEEE 2nd International Conference on Advanced Trends in Information Theory (ATIT), 2020, pp. 330–336, doi: https://doi.org/10.1109/ATIT50783.2020.9349321.
- S. Piltyay, A. Bulashenko, H. Kushnir, O. Bulashenko, “New tunable iris-post square waveguide polarizers for satellite information systems,” in 2020 IEEE 2nd International Conference on Advanced Trends in Information Theory (ATIT), 2020, pp. 342–348, doi: https://doi.org/10.1109/ATIT50783.2020.9349357.
- J. Helszajn, Microwave Polarizers, Power Dividers, Phase Shifters, Circulators, and Switches. Wiley, 2018, doi: https://doi.org/10.1002/9781119490104.
- S. A. Maas, Practical Microwave Circuits. Norwood: Artech House, 2014, uri: https://us.artechhouse.com/Practical-Microwave-Circuits-P1659.aspx.
- D. M. Pozar, Microwave Engineering, 4th ed. New Jersey: Wiley and Sons, 2011, uri: https://www.wiley.com/en-us/Microwave+Engineering%2C+4th+Edition-p-9780470631553.
- R. Sorrentino, G. Bianchi, Microwave and RD Engineering. New Jersey: John Wiley & Sons, 2010, uri: https://www.wiley.com/en-us/Microwave+and+RF+Engineering-p-9780470660218.
- N. Marcuvitz, Waveguide Handbook. Short Run Press Ltd., 1986.
- R. E. Collin, Foundations for Microwave Engineering. Wiley-IEEE Press, 2001, uri: https://ieeexplore.ieee.org/book/5265446.
- R. E. Collin, Field Theory of Guided Waves, 2nd ed. Wiley-IEEE Press, 1990, uri: https://www.wiley.com/en-us/Field+Theory+of+Guided+Waves%2C+2nd+Edition-p-9780879422370.
- W. L. Stutzman, Polarization in Electromagnetic Systems, 2nd ed. Artech House, 2018, uri: https://us.artechhouse.com/Polarization-in-Electromagnetic-Systems-Second-Edition-P1945.aspx.