Inverse problem of triplet BPF with all mixed couplings
DOI:
https://doi.org/10.3103/S0735272724050017Keywords:
bandpass filter, mixed coupling, frequency responses, transmission zero, group delayAbstract
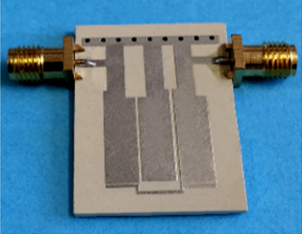
In this article, using the inverse problem method, an analysis of a third-order bandpass filter (triplet BPF) with all mixed couplings was carried out. These filters have advantages over simpler triplet BPFs with partially used mixed couplings. The inverse problem method determines the mixed coupling coefficients of a triplet BPF from a given location of two transmission zeros (TZ) on the complex plane s = σ + jΩ. As a result of the analysis, it was found that triplet BPFs with all mixed couplings more effectively bring together TZs located on the σ or jΩ axis, which leads to an increase of flat group delay or BPF selectivity. Microstrip triplet BPFs with all mixed couplings use stepped-impedance resonators (SIR) located a short distance from each other. This results in very compact filters formed according to the “close-packed” principle. In such microstrip filters, increasing the thickness of the dielectric substrate makes it possible to increase the unloaded quality factor Qu of the resonator and realize narrow passbands without increasing the area occupied by the filter. A universal microstrip triplet BPF with all mixed couplings is considered. It combines the properties of symmetric and asymmetric triplet BPF and provides 10 different locations of two TZs on the s-plane and forms significant variety of frequency responses.
References
- G. L. Matthaei, L. Young, E. M. T. Jones, Microwave Filters, Impedance-Matching Networks, and Coupling Structures. New York: Artech House Books, 1980.
- J.-S. Hong, Microstrip Filters for RF/Microwave Applications, 2nd ed. New Jersey: Wiley, 2011, doi: https://doi.org/10.1002/9780470937297.
- R. J. Cameron, C. M. Kudsia, R. R. Mansour, Microwave Filters for Communication Systems, 2nd ed. Hoboken, NJ: John Wiley & Sons, Inc., 2018, doi: https://doi.org/10.1002/9781119292371.
- A. V. Zakharov, S. M. Litvintsev, “Lumped-distributed resonators providing N or 2N transmission zeros at real frequencies in bandpass filters without cross and mixed couplings,” IEEE Trans. Microw. Theory Tech., vol. 72, no. 6, pp. 3682–3691, 2024, doi: https://doi.org/10.1109/TMTT.2023.3332260.
- A. V. Zakharov, S. M. Litvintsev, “Lumped-distributed resonators providing multiple transmission zeros in bandpass filters with simple and mixed couplings,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 71, no. 8, pp. 3502–3513, 2024, doi: https://doi.org/10.1109/TCSI.2024.3375961.
- X. Wu, Y. Cao, B. Yuan, Y. Qi, G. Wang, “Bandpass filters using single and cascaded novel triple-mode ceramic monoblocks,” IEEE Trans. Components, Packag. Manuf. Technol., vol. 13, no. 7, pp. 965–977, 2023, doi: https://doi.org/10.1109/TCPMT.2023.3296108.
- Y. Wu, K. Ma, “Design of multiband bandpass inline topology filters using compact multicoupled line structures,” IEEE Trans. Components, Packag. Manuf. Technol., vol. 13, no. 3, pp. 382–390, 2023, doi: https://doi.org/10.1109/TCPMT.2023.3256977.
- A. Zakharov, M. Ilchenko, “Circuit function characterizing tunability of resonators,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 67, no. 1, pp. 98–107, 2020, doi: https://doi.org/10.1109/TCSI.2019.2940066.
- A. V. Zakharov, M. E. Il’chenko, “A new approach to designing varicap-tuned filters,” J. Commun. Technol. Electron., vol. 55, no. 12, pp. 1424–1431, 2010, doi: https://doi.org/10.1134/S1064226910120156.
- A. Fukasawa, “Analysis and composition of a new microwave filter configuration with inhomogeneous dielectric medium,” IEEE Trans. Microw. Theory Tech., vol. 30, no. 9, pp. 1367–1375, 1982, doi: https://doi.org/10.1109/TMTT.1982.1131262.
- L. K. Yeung, K.-L. Wu, Y. E. Wang, “Low-temperature cofired ceramic LC filters for RF applications [Applications Notes],” IEEE Microw. Mag., vol. 9, no. 5, pp. 118–128, 2008, doi: https://doi.org/10.1109/MMM.2008.927634.
- T. Ishizaki, M. Fujita, H. Kagata, T. Uwano, H. Miyake, “A very small dielectric planar filter for portable telephones,” in 1993 IEEE MTT-S International Microwave Symposium Digest, 1993, pp. 177–180, doi: https://doi.org/10.1109/MWSYM.1993.276916.
- A. V. Zakharov, S. A. Rozenko, N. A. Zakharova, “Microstrip bandpass filters on substrates with high permittivities,” J. Commun. Technol. Electron., vol. 57, no. 3, pp. 342–351, 2012, doi: https://doi.org/10.1134/S1064226912020143.
- A. V. Zakharov, S. M. Litvintsev, “Coupling matrix modification for bandpass filters with through-type resonators and simple couplings,” IEEE Trans. Circuits Syst. II Express Briefs, vol. 71, no. 4, pp. 1864–1868, 2024, doi: https://doi.org/10.1109/TCSII.2023.3337439.
- A. Zakharov, S. Litvintsev, “Expanding functionality of dual-mode resonators and filters using nonuniform transmission line structural elements,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 69, no. 8, pp. 3124–3135, 2022, doi: https://doi.org/10.1109/TCSI.2022.3169472.
- D. Morgan, Surface Acoustic Wave Filters: With Applications to Electronic Communications and Signal Processing. Academic Press, 2010.
- M. Makimoto, S. Yamashita, Microwave Resonators and Filters for Wireless Communication, vol. 4. Berlin, Heidelberg: Springer Berlin Heidelberg, 2001, doi: https://doi.org/10.1007/978-3-662-04325-7.
- A. Zakharov, M. Ilchenko, “Coupling coefficients between resonators in stripline combline and pseudocombline bandpass filters,” IEEE Trans. Microw. Theory Tech., vol. 68, no. 7, pp. 2679–2690, 2020, doi: https://doi.org/10.1109/TMTT.2020.2988866.
- A. V. Zakharov, M. Y. Ilchenko, L. S. Pinchuk, “Coupling coefficient of quarter-wave resonators as a function of parameters of comb stripline filters,” Radioelectron. Commun. Syst., vol. 58, no. 6, pp. 284–289, 2015, doi: https://doi.org/10.3103/S0735272715060060.
- A. V. Zakharov, “Stripline combline filters on substrates designed on high-permittivity ceramic materials,” J. Commun. Technol. Electron., vol. 58, no. 3, pp. 265–272, 2013, doi: https://doi.org/10.1134/S1064226913030145.
- S. Amari, M. Bekheit, F. Seyfert, “Notes on bandpass filters whose inter-resonator coupling coefficients are linear functions of frequency,” in 2008 IEEE MTT-S International Microwave Symposium Digest, 2008, pp. 1207–1210, doi: https://doi.org/10.1109/MWSYM.2008.4633275.
- L. Szydlowski, A. Lamecki, M. Mrozowski, “Coupled-resonator filters with frequency-dependent couplings: Coupling matrix synthesis,” IEEE Microw. Wirel. Components Lett., vol. 22, no. 6, pp. 312–314, 2012, doi: https://doi.org/10.1109/LMWC.2012.2197386.
- L. Szydlowski, N. Leszczynska, M. Mrozowski, “A linear phase filter in quadruplet topology with frequency-dependent couplings,” IEEE Microw. Wirel. Components Lett., vol. 24, no. 1, pp. 32–34, 2014, doi: https://doi.org/10.1109/LMWC.2013.2288178.
- S. Tamiazzo, G. Macchiarella, “Synthesis of cross-coupled filters with frequency-dependent couplings,” IEEE Trans. Microw. Theory Tech., vol. 65, no. 3, pp. 775–782, 2017, doi: https://doi.org/10.1109/TMTT.2016.2633258.
- Q.-X. Chu, H. Wang, “A compact open-loop filter with mixed electric and magnetic coupling,” IEEE Trans. Microw. Theory Tech., vol. 56, no. 2, pp. 431–439, 2008, doi: https://doi.org/10.1109/TMTT.2007.914642.
- H. Wang, Q.-X. Chu, “An inline coaxial quasi-elliptic filter with controllable mixed electric and magnetic coupling,” IEEE Trans. Microw. Theory Tech., vol. 57, no. 3, pp. 667–673, 2009, doi: https://doi.org/10.1109/TMTT.2009.2013290.
- F. Zhu, W. Hong, J.-X. Chen, K. Wu, “Quarter-wavelength stepped-impedance resonator filter with mixed electric and magnetic coupling,” IEEE Microw. Wirel. Components Lett., vol. 24, no. 2, pp. 90–92, 2014, doi: https://doi.org/10.1109/LMWC.2013.2290225.
- R. Levy, “New cascaded trisections with resonant cross-couplings (CTR Sections) applied to the design of optimal filters,” in IEEE MTT-S International Microwave Symposium Digest, 2004, vol. 2, pp. 447–450, doi: https://doi.org/10.1109/mwsym.2004.1336007.
- K. Gong, W. Hong, Y. Zhang, P. Chen, C. J. You, “Substrate integrated waveguide quasi-elliptic filters with controllable electric and magnetic mixed coupling,” IEEE Trans. Microw. Theory Tech., vol. 60, no. 10, pp. 3071–3078, 2012, doi: https://doi.org/10.1109/TMTT.2012.2209437.
- W. Shen, L.-S. Wu, X.-W. Sun, W.-Y. Yin, J.-F. Mao, “Novel substrate integrated waveguide filters with mixed cross coupling (MCC),” IEEE Microw. Wirel. Components Lett., vol. 19, no. 11, pp. 701–703, 2009, doi: https://doi.org/10.1109/LMWC.2009.2032007.
- L. Szydlowski, A. Jedrzejewski, M. Mrozowski, “A trisection filter design with negative slope of frequency-dependent crosscoupling implemented in substrate integrated waveguide (SIW),” IEEE Microw. Wirel. Components Lett., vol. 23, no. 9, pp. 456–458, 2013, doi: https://doi.org/10.1109/LMWC.2013.2272611.
- A. Zakharov, “Transmission zeros of trisection and quadruplet bandpass filters with mixed cross coupling,” IEEE Trans. Microw. Theory Tech., vol. 69, no. 1, pp. 89–100, 2021, doi: https://doi.org/10.1109/TMTT.2020.3034663.
- A. Zakharov, S. Litvintsev, M. Ilchenko, “Trisection bandpass filters with all mixed couplings,” IEEE Microw. Wirel. Components Lett., vol. 29, no. 9, pp. 592–594, 2019, doi: https://doi.org/10.1109/LMWC.2019.2929650.
- G. A. Korn, T. M. Korn, Mathematical Handbook for Scientists and Engineers: Definitions, Theorems, and Formulas for Reference and Review. New York, NY: McGraw-Hill, 1961.
- P. Zhao, K. Wu, “Cascading fundamental building blocks with frequency-dependent couplings in microwave filters,” IEEE Trans. Microw. Theory Tech., vol. 67, no. 4, pp. 1432–1440, 2019, doi: https://doi.org/10.1109/TMTT.2019.2895532.
- Y. Zhang, F. Seyfert, S. Amari, M. Olivi, K.-L. Wu, “General synthesis method for dispersively coupled resonator filters with cascaded topologies,” IEEE Trans. Microw. Theory Tech., vol. 69, no. 2, pp. 1378–1393, 2021, doi: https://doi.org/10.1109/TMTT.2020.3041223.