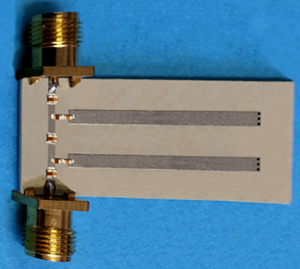
Bandpass filters with increased to 3N+1 number of attenuation poles

Sergii Litvintsev; Alexander Zakharov

doi:10.3103/S073527272311002X

Authors

Sergii Litvintsev National Technical University of Ukraine “Igor Sikorsky Kyiv Polytechnic Institute”, Kyiv, Ukraine https://orcid.org/0000-0002-6171-0036
Alexander Zakharov National Technical University of Ukraine “Igor Sikorsky Kyiv Polytechnic Institute”, Kyiv, Ukraine https://orcid.org/0000-0002-1222-1623

DOI:

https://doi.org/10.3103/S073527272311002X

Keywords:

bandpass filter, frequency response, transmission zero, attenuation pole, lumped-distributed resonator, input admittance, resonant frequency, anti-resonant frequency

Abstract

N-order bandpass filter (BPF) with parallel resonators and cross and mixed couplings can have (N + 1) transmission zeros (TZ) located on the complex plane s = σ + jω. TZs at real frequencies (jω axis) are also called attenuation poles (AP). The authors propose an alternative possibility of AP forming in filters using resonators with special properties, which significantly increases the AP number from (N + 1) to (3N + 1). Increasing the AP number with fewer resonators allows us to increase the selectivity and rejection level and reduce BPF insertion losses. The special properties of resonators are that their input admittance Y has one or two poles (ω_p1, ω_p2) located next to the resonance frequency ω₀. This leads to the appearance of AP in a BPF. We propose and analyze three resonators with special properties. They are formed by a cascade connection of a quarter-wave resonator and lumped L and C. The input of the resonators is located on the side of the lumped elements. It was found that the Q-factors of lumped elements do not affect the filter losses in the passband. The reduction of Q_L and Q_C leads only to the decrease of the AP “depth.” For the first time, 7 APs were implemented in the experimental second-order microstrip BPF.

References

M. Malki, L. Yang, R. Gomez-Garcia, “Input-reflectionless quasi-elliptic-type single- and dual-band bandpass filters based on passive channelized principles,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 70, no. 1, pp. 190–202, 2023, doi: https://doi.org/10.1109/TCSI.2022.3207880.

A. V. Zakharov, S. M. Litvintsev, “Lumped-distributed resonators providing N or 2N transmission zeros at real frequencies in bandpass filters without cross and mixed couplings,” IEEE Trans. Microw. Theory Tech., vol. 72, no. 6, pp. 3682–3691, 2024, doi: https://doi.org/10.1109/TMTT.2023.3332260.

A. V. Zakharov, S. M. Litvintsev, “Coupling matrix modification for bandpass filters with through-type resonators and simple couplings,” IEEE Trans. Circuits Syst. II Express Briefs, vol. 71, no. 4, pp. 1864–1868, 2024, doi: https://doi.org/10.1109/TCSII.2023.3337439.

J. Lee, J. Lee, “Transmission-line bandpass filter structures with infinite reflectionless range,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 69, no. 6, pp. 2387–2398, 2022, doi: https://doi.org/10.1109/TCSI.2022.3157323.

A. V. Zakharov, S. M. Litvintsev, “Lumped-distributed resonators providing multiple transmission zeros in bandpass filters with simple and mixed couplings,” IEEE Trans. Circuits Syst. I Regul. Pap., pp. 1–12, 2024, doi: https://doi.org/10.1109/TCSI.2024.3375961.

D. Morgan, Surface Acoustic Wave Filters: With Applications to Electronic Communications and Signal Processing. Academic Press, 2010.

A. V. Zakharov, S. A. Rozenko, N. A. Zakharova, “Microstrip bandpass filters on substrates with high permittivities,” J. Commun. Technol. Electron., vol. 57, no. 3, pp. 342–351, 2012, doi: https://doi.org/10.1134/S1064226912020143.

A. V. Zakharov, M. E. Il’сhenko, V. N. Korpach, “Features of the coupling coefficients of planar stepped-impedance resonators at higher resonance frequencies and application of such resonators for suppression of spurious passbands,” J. Commun. Technol. Electron., vol. 59, no. 6, pp. 550–556, 2014, doi: https://doi.org/10.1134/S1064226914060217.

A. Zakharov, M. Ilchenko, “Trisection microstrip delay line filter with mixed cross-coupling,” IEEE Microw. Wirel. Components Lett., vol. 27, no. 12, pp. 1083–1085, 2017, doi: https://doi.org/10.1109/LMWC.2017.2759724.

M. Makimoto, S. Yamashita, Microwave Resonators and Filters for Wireless Communication, vol. 4. Berlin, Heidelberg: Springer Berlin Heidelberg, 2001, doi: https://doi.org/10.1007/978-3-662-04325-7.

A. Fukasawa, “Analysis and composition of a new microwave filter configuration with inhomogeneous dielectric medium,” IEEE Trans. Microw. Theory Tech., vol. 30, no. 9, pp. 1367–1375, 1982, doi: https://doi.org/10.1109/TMTT.1982.1131262.

L. K. Yeung, K.-L. Wu, Y. E. Wang, “Low-temperature cofired ceramic LC filters for RF applications [Applications Notes],” IEEE Microw. Mag., vol. 9, no. 5, pp. 118–128, 2008, doi: https://doi.org/10.1109/MMM.2008.927634.

T. Ishizaki, M. Fujita, H. Kagata, T. Uwano, H. Miyake, “A very small dielectric planar filter for portable telephones,” in 1993 IEEE MTT-S International Microwave Symposium Digest, 1993, pp. 177–180, doi: https://doi.org/10.1109/MWSYM.1993.276916.

A. V. Zakharov, M. Y. Ilchenko, L. S. Pinchuk, “Coupling coefficient of quarter-wave resonators as a function of parameters of comb stripline filters,” Radioelectron. Commun. Syst., vol. 58, no. 6, pp. 284–289, 2015, doi: https://doi.org/10.3103/S0735272715060060.

A. V. Zakharov, M. E. Il’chenko, “Pseudocombline bandpass filters based on half-wave resonators manufactured from sections of balanced striplines,” J. Commun. Technol. Electron., vol. 60, no. 7, pp. 801–807, 2015, doi: https://doi.org/10.1134/S1064226915060182.

A. V. Zakharov, “Stripline combline filters on substrates designed on high-permittivity ceramic materials,” J. Commun. Technol. Electron., vol. 58, no. 3, pp. 265–272, 2013, doi: https://doi.org/10.1134/S1064226913030145.

A. E. Atia, A. E. Williams, “Narrow-bandpass waveguide filters,” IEEE Trans. Microw. Theory Tech., vol. 20, no. 4, pp. 258–265, 1972, doi: https://doi.org/10.1109/TMTT.1972.1127732.

A. Atia, A. Williams, R. Newcomb, “Narrow-band multiple-coupled cavity synthesis,” IEEE Trans. Circuits Syst., vol. 21, no. 5, pp. 649–655, 1974, doi: https://doi.org/10.1109/TCS.1974.1083913.

R. J. Cameron, C. M. Kudsia, R. R. Mansour, Microwave Filters for Communication Systems, 2nd ed. Hoboken, NJ: John Wiley & Sons, Inc., 2018, doi: https://doi.org/10.1002/9781119292371.

S. Amari, U. Rosenberg, J. Bornemann, “Adaptive synthesis and design of resonator filters with source/load-multiresonator coupling,” IEEE Trans. Microw. Theory Tech., vol. 50, no. 8, pp. 1969–1978, 2002, doi: https://doi.org/10.1109/TMTT.2002.801348.

R. Levy, “New cascaded trisections with resonant cross-couplings (CTR Sections) applied to the design of optimal filters,” in IEEE MTT-S International Microwave Symposium Digest, 2004, vol. 2, pp. 447–450, doi: https://doi.org/10.1109/mwsym.2004.1336007.

K. Ma, J.-G. Ma, K. S. Yeo, M. A. Do, “A compact size coupling controllable filter with separate electric and magnetic coupling paths,” IEEE Trans. Microw. Theory Tech., vol. 54, no. 3, pp. 1113–1119, 2006, doi: https://doi.org/10.1109/TMTT.2005.864118.

Q.-X. Chu, H. Wang, “A compact open-loop filter with mixed electric and magnetic coupling,” IEEE Trans. Microw. Theory Tech., vol. 56, no. 2, pp. 431–439, 2008, doi: https://doi.org/10.1109/TMTT.2007.914642.

S. Amari, M. Bekheit, F. Seyfert, “Notes on bandpass filters whose inter-resonator coupling coefficients are linear functions of frequency,” in 2008 IEEE MTT-S International Microwave Symposium Digest, 2008, pp. 1207–1210, doi: https://doi.org/10.1109/MWSYM.2008.4633275.

S. Tamiazzo, G. Macchiarella, “Synthesis of cross-coupled filters with frequency-dependent couplings,” IEEE Trans. Microw. Theory Tech., vol. 65, no. 3, pp. 775–782, 2017, doi: https://doi.org/10.1109/TMTT.2016.2633258.

J. J. Vague et al., “Inline combline filters of order N with up to N + 1 transmission zeros,” IEEE Trans. Microw. Theory Tech., vol. 69, no. 7, pp. 3287–3297, 2021, doi: https://doi.org/10.1109/TMTT.2021.3072370.

M. Mul, M. Jasinski, A. Lamecki, R. Gomez-Garcia, M. Mrozowski, “Inline microwave filters with N+1 transmission zeros generated by frequency-variant couplings: coupling-matrix-based synthesis and design,” IEEE Trans. Circuits Syst. II Express Briefs, vol. 69, no. 3, pp. 824–828, 2022, doi: https://doi.org/10.1109/TCSII.2021.3125460.

G. L. Matthaei, L. Young, E. M. T. Jones, Microwave Filters, Impedance-Matching Networks, and Coupling Structures. New York: Artech House Books, 1980.

C.-J. Chen, “A coupled-line coupling structure for the design of quasi-elliptic bandpass аilters,” IEEE Trans. Microw. Theory Tech., vol. 66, no. 4, pp. 1921–1925, 2018, doi: https://doi.org/10.1109/TMTT.2017.2783378.

H. Wang, Q.-X. Chu, “An inline coaxial quasi-elliptic filter with controllable mixed electric and magnetic coupling,” IEEE Trans. Microw. Theory Tech., vol. 57, no. 3, pp. 667–673, 2009, doi: https://doi.org/10.1109/TMTT.2009.2013290.

AVX, “RF/Microwave Products, AVX Corporation,” Products. https://www.kyocera-avx.com/products/.

A. V. Zakharov, M. Y. Ilchenko, V. Y. Karnauh, L. S. Pinchuk, “Stripline bandpass filters with step-impedance resonators,” Radioelectron. Commun. Syst., vol. 54, no. 3, pp. 163–169, 2011, doi: https://doi.org/10.3103/S0735272711030071.

A. Zakharov, “Parametric and structural-parametric synthesis of nonuniform transmission line resonators,” IEEE Trans. Circuits Syst. I Regul. Pap., vol. 68, no. 3, pp. 1055–1067, 2021, doi: https://doi.org/10.1109/TCSI.2020.3044925.

A. V. Zakharov, M. E. Il’chenko, “Thin bandpass filters containing sections of symmetric strip transmission line,” J. Commun. Technol. Electron., vol. 58, no. 7, pp. 728–736, 2013, doi: https://doi.org/10.1134/S1064226913060144.

C. Wang, K. A. Zaki, A. E. Atia, T. G. Dolan, “Dielectric combline resonators and filters,” IEEE Trans. Microw. Theory Tech., vol. 46, no. 12, pp. 2501–2506, 1998, doi: https://doi.org/10.1109/22.739240.

C. Wang, K. Zaki, “Dielectric resonators and filters,” IEEE Microw. Mag., vol. 8, no. 5, pp. 115–127, 2007, doi: https://doi.org/10.1109/MMM.2007.903648.