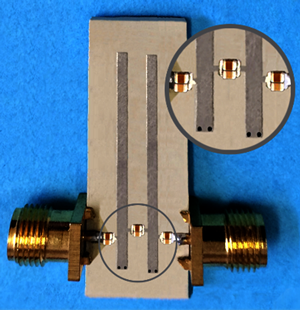
Bandpass filters with 2N+1 transmission zeros at real frequencies

Sergii Litvintsev; Alexander Zakharov

doi:10.3103/S0735272723100035

Authors

Sergii Litvintsev Igor Sikorsky Kyiv Polytechnic Institute, Kyiv, Ukraine https://orcid.org/0000-0002-6171-0036
Alexander Zakharov Igor Sikorsky Kyiv Polytechnic Institute, Kyiv, Ukraine https://orcid.org/0000-0002-1222-1623

DOI:

https://doi.org/10.3103/S0735272723100035

Keywords:

bandpass filter, cross-coupling, frequency response, resonant frequency, transmission zero

Abstract

This paper discusses N-order bandpass filter (BPF) with all mixed couplings between adjacent resonators, and between end resonators and loads. It has (2N +1) transmission zeros (TZ) at real frequencies. To the existing (N +1) TZs generated by mixed couplings, another N TZs are added, which generated by resonators with special properties. Each such resonator is a λ/4 resonator, in which the connection point is located relatively close to the short-circuited end. In this case, the input admittance of the resonator Y(jω) has a pole ω_p, which is located to the right and at a small distance from the main resonant frequency ω₀. This pole ω_p of the Y(jω) function leads to the appearance of a right-handed TZ in a BPF characteristic. If we use N resonators with different connection points, then N additional TZs will appear. The design of that BPF is considered. The measured and simulated frequency responses of the second-order BPF with 5 TZs are presented.

References

G. L. Matthaei, L. Young, E. M. T. Jones, Microwave Filters, Impedance-Matching Networks, and Coupling Structures. New York: Artech House Books, 1980.

J.-S. Hong, Microstrip Filters for RF/Microwave Applications, 2nd ed. New Jersey: Wiley, 2011, doi: https://doi.org/10.1002/9780470937297.

X. Wu, Y. Cao, B. Yuan, Y. Qi, G. Wang, “Bandpass filters using single and cascaded novel triple-mode ceramic monoblocks,” IEEE Trans. Components, Packag. Manuf. Technol., vol. 13, no. 7, pp. 965–977, 2023, doi: https://doi.org/10.1109/TCPMT.2023.3296108.

Y. Wu, K. Ma, “Design of multiband bandpass inline topology filters using compact multicoupled line structures,” IEEE Trans. Components, Packag. Manuf. Technol., vol. 13, no. 3, pp. 382–390, 2023, doi: https://doi.org/10.1109/TCPMT.2023.3256977.

A. V. Zakharov, S. M. Litvintsev, “Coupling matrix modification for bandpass filters with through-type resonators and simple couplings,” IEEE Trans. Circuits Syst. II Express Briefs, pp. 1–1, 2023, doi: https://doi.org/10.1109/TCSII.2023.3337439.

A. V. Zakharov, S. M. Litvintsev, “Lumped-distributed resonators providing N or 2N transmission zeros at real frequencies in bandpass filters without cross and mixed couplings,” IEEE Trans. Microw. Theory Tech., pp. 1–10, 2023, doi: https://doi.org/10.1109/TMTT.2023.3332260.

R. M. Kurzrok, “General three-resonator filters in waveguide,” IEEE Trans. Microw. Theory Tech., vol. 14, no. 1, pp. 46–47, 1966, doi: https://doi.org/10.1109/TMTT.1966.1126154.

R. M. Kurzrok, “General four-resonator filters at microwave frequencies,” IEEE Trans. Microw. Theory Tech., vol. MTT-14, no. 6, pp. 295–296, 1966, doi: https://doi.org/10.1109/TMTT.1966.1126254.

A. Atia, A. Williams, R. Newcomb, “Narrow-band multiple-coupled cavity synthesis,” IEEE Trans. Circuits Syst., vol. 21, no. 5, pp. 649–655, 1974, doi: https://doi.org/10.1109/TCS.1974.1083913.

R. J. Cameron, C. M. Kudsia, R. R. Mansour, Microwave Filters for Communication Systems, 2nd ed. Hoboken, NJ: John Wiley & Sons, Inc., 2018, doi: https://doi.org/10.1002/9781119292371.

R. A. Johnson, Mechanical Filters in Electronics. John Wiley & Sons, 1983, uri: https://www.amazon.com/Mechanical-Filters-Electronics-Wiley-filters/dp/0471089192.

K. Ma, J.-G. Ma, K. S. Yeo, M. A. Do, “A compact size coupling controllable filter with separate electric and magnetic coupling paths,” IEEE Trans. Microw. Theory Tech., vol. 54, no. 3, pp. 1113–1119, 2006, doi: https://doi.org/10.1109/TMTT.2005.864118.

A. Zakharov, “Transmission zeros of trisection and quadruplet bandpass filters with mixed cross coupling,” IEEE Trans. Microw. Theory Tech., vol. 69, no. 1, pp. 89–100, 2021, doi: https://doi.org/10.1109/TMTT.2020.3034663.

S. Amari, M. Bekheit, F. Seyfert, “Notes on bandpass filters whose inter-resonator coupling coefficients are linear functions of frequency,” in 2008 IEEE MTT-S International Microwave Symposium Digest, 2008, pp. 1207–1210, doi: https://doi.org/10.1109/MWSYM.2008.4633275.

L. Szydlowski, N. Leszczynska, M. Mrozowski, “Generalized Chebyshev bandpass filters with frequency-dependent couplings based on stubs,” IEEE Trans. Microw. Theory Tech., vol. 61, no. 10, pp. 3601–3612, 2013, doi: https://doi.org/10.1109/TMTT.2013.2279777.

R. Levy, “New cascaded trisections with resonant cross-couplings (CTR Sections) applied to the design of optimal filters,” in IEEE MTT-S International Microwave Symposium Digest, 2004, vol. 2, pp. 447–450, doi: https://doi.org/10.1109/mwsym.2004.1336007.

J. J. Vague et al., “Inline combline filters of order N with up to N + 1 transmission zeros,” IEEE Trans. Microw. Theory Tech., vol. 69, no. 7, pp. 3287–3297, 2021, doi: https://doi.org/10.1109/TMTT.2021.3072370.

M. Mul, M. Jasinski, A. Lamecki, R. Gomez-Garcia, M. Mrozowski, “Inline microwave filters with N+1 transmission zeros generated by frequency-variant couplings: coupling-matrix-based synthesis and design,” IEEE Trans. Circuits Syst. II Express Briefs, vol. 69, no. 3, pp. 824–828, 2022, doi: https://doi.org/10.1109/TCSII.2021.3125460.

A. Zakharov, M. Ilchenko, “Trisection microstrip delay line filter with mixed cross-coupling,” IEEE Microw. Wirel. Components Lett., vol. 27, no. 12, pp. 1083–1085, 2017, doi: https://doi.org/10.1109/LMWC.2017.2759724.

A. Zakharov, S. Litvintsev, “Transmission zeros in bandpass filters with simple and Mixed Couplings,” IEEE Trans. Circuits Syst. I Fundam. Theory Appl., 2024, doi: https://doi.org/10.1109/TCSI.2024.3375961.

P. Zero, “Tunable Filter MAXI-POLE datasheet,” 2017. https://www.mpgdover.com/content/dam/mpg/documents/datasheets/filters/band-pass/tunable/combline/MAXI-POLE Datasheet.pdf.