Forced lateral vibrations of magnetically soft microribbon: Construction of partial solutions

Andrii Sizhuk; Zhenjie Zhao; Xiaohong Chen; Zhuo Sun; Guangjiong Dong; Oleksandr Prokopenko; Alina Tretyak

doi:10.3103/S0735272723080046

Authors

Andrii Sizhuk Taras Shevchenko National University of Kyiv, Kyiv, Ukraine https://orcid.org/0000-0002-8522-7620
Zhenjie Zhao East China Normal University, Shanghai, China https://orcid.org/0000-0001-5366-4896
Xiaohong Chen East China Normal University, Shanghai, China
Zhuo Sun East China Normal University, Shanghai, China
Guangjiong Dong East China Normal University, Shanghai, China
Oleksandr Prokopenko Taras Shevchenko National University of Kyiv, Kyiv, Ukraine https://orcid.org/0000-0002-4378-0866
Alina Tretyak Lesya Ukrainka Volyn National University, Lutsk, Ukraine https://orcid.org/0000-0002-1776-5339

DOI:

https://doi.org/10.3103/S0735272723080046

Keywords:

sound vibrations, magnetically soft, microribbon, FINEMET, magnetic field

Abstract

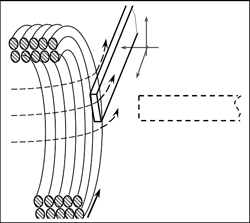
The mathematical description of low-frequency vibrations of the magnetically soft precision microribbon Fe_73.5Cu₁Nb₃Si_13.5B₉ with the fixed one end is proposed in the paper. Resonant frequencies of induced mechanical vibrations of microribbons are found in the given frequency range of 15–80 Hz, for an alternating magnetic field. The attenuation coefficient, measured for the mechanical self-vibration of the annealed sample, is derived. This coefficient measured by laser turns out to be much smaller than the corresponding half-width of the amplitude-frequency characteristic (frequency response) of the described above forced vibrations. The theoretical model of forced and free microribbon vibrations with finite thickness and width is proposed. One of the created partial solutions properties is a relatively wide resonance curve, which is confirmed experimentally. The given examples of partial solutions confirm the assumption about the frequency response shape below the maximum frequency at the specified excitation frequency and system parameters. The dependence of the mechanical parameter characterizing the force moment during bending deformation on the applied magnetic field intensity determines the mechanical properties of soft magnetic crystalline materials.

References

W. Martienssen, H. Warlimont, Springer Handbook of Condensed Matter and Materials Data. Berlin: Springer Berlin Heidelberg, 2005, doi: https://doi.org/10.1007/3-540-30437-1.

J.-M. Le Breton, A. Zorkovská, M. Kasiarová, “Crystallization of Fe 73.5 Cu 1 RE 3 Si 13.5 B 9 ribbons with RE = Pr, Nd, Gd,” J. Phys. Condens. Matter, vol. 16, no. 30, pp. 5555–5568, 2004, doi: https://doi.org/10.1088/0953-8984/16/30/016.

Y. Yoshizawa, S. Oguma, K. Yamauchi, “New Fe-based soft magnetic alloys composed of ultrafine grain structure,” J. Appl. Phys., vol. 64, no. 10, pp. 6044–6046, 1988, doi: https://doi.org/10.1063/1.342149.

Y. Yoshizawa, K. Yamauchi, “Magnetic properties of Fe Cu M Si B (M = Cr, V, Mo, Nb, Ta, W) alloys,” Mater. Sci. Eng. A, vol. 133, pp. 176–179, 1991, doi: https://doi.org/10.1016/0921-5093(91)90043-M.

M. Tejedor et al., “Magnetoimpedance effect in zero magnetostriction nanocrystalline Fe73.5Cu1Nb3Si16.5B6 ribbons,” J. Magn. Magn. Mater., vol. 185, no. 1, pp. 61–65, 1998, doi: https://doi.org/10.1016/S0304-8853(98)00005-5.

A. V. Chumak, V. I. Vasyuchka, A. A. Serga, B. Hillebrands, “Magnon spintronics,” Nat. Phys., vol. 11, no. 6, pp. 453–461, 2015, doi: https://doi.org/10.1038/nphys3347.

D. R. Askeland, The Science and Engineering of Materials. Boston, MA: Springer US, 1996, doi: https://doi.org/10.1007/978-1-4899-2895-5.

D. G. Rethwisch, W. D. Callister Jr., Materials Science and Engineering: An Introduction, 10th Edition. New Jersey: Wiley, 2018, uri: https://www.wiley.com/en-us/Materials+Science+and+Engineering%3A+An+Introduction%2C+10th+Edition-p-9781119405498.

J. Xie, Y.-F. Liao, D.-N. Wu, W.-J. Xiao, Q. Xie, “The degree‐of‐order dependent electronic structures and magnetic properties of Fe 3 Si alloys,” Phys. Status Solidi, vol. 257, no. 5, 2020, doi: https://doi.org/10.1002/pssb.201900667.

T. Xu, J. Zhang, M. Salehizadeh, O. Onaizah, E. Diller, “Millimeter-scale flexible robots with programmable three-dimensional magnetization and motions,” Sci. Robot., vol. 4, no. 29, 2019, doi: https://doi.org/10.1126/scirobotics.aav4494.

E. E. Niedert et al., “A tumbling magnetic microrobot system for biomedical applications,” Micromachines, vol. 11, no. 9, p. 861, 2020, doi: https://doi.org/10.3390/mi11090861.

X. Yan et al., “Multifunctional biohybrid magnetite microrobots for imaging-guided therapy,” Sci. Robot., vol. 2, no. 12, 2017, doi: https://doi.org/10.1126/scirobotics.aaq1155.

T. M. Wallis, J. Moreland, P. Kabos, “Einstein–de Haas effect in a NiFe film deposited on a microcantilever,” Appl. Phys. Lett., vol. 89, no. 12, 2006, doi: https://doi.org/10.1063/1.2355445.

A. I. Ahiezer, V. G. Barikhtar, S. V. Peletminckii, Spin Waves. Moscow: Nauka, 1967.

Y. Kobljanskyj et al., “FINEMET micro-ribbons: The experimental identification of the object,” in Springer Proceedings in Physics, Cham: Springer, 2021, pp. 33–54.

S. P. Timoshenko, D. H. Yang, U. Uiver, Kolebaniya v Inzhenernom Dele. Moscow: Mashinostrojenie, 1985.

A. Sizhuk et al., “The vibration of FINEMET microribbon in magnetic field at low sound frequency,” in 2022 IEEE 2nd Ukrainian Microwave Week (UkrMW), 2022, pp. 410–414, doi: https://doi.org/10.1109/UkrMW58013.2022.10037134.

G. Huang, B. Li, “The combined magnetic-vibration stress relief,” Results Phys., vol. 47, p. 106372, 2023, doi: https://doi.org/10.1016/j.rinp.2023.106372.

G. Ouyang, X. Chen, Y. Liang, C. Macziewski, J. Cui, “Review of Fe-6.5 wt%Si high silicon steel—A promising soft magnetic material for sub-kHz application,” J. Magn. Magn. Mater., vol. 481, pp. 234–250, 2019, doi: https://doi.org/10.1016/j.jmmm.2019.02.089.

T. Gavasheli, G. I. Mamniashvili, G. Ghvedashvili, T. O. Gegechkori, “NMR-spin-echo study of pinning of domain walls in cobalt micropowders, nanopowders and nanowires,” Radioelectron. Commun. Syst., vol. 66, no. 9, p. 481, 2023, doi: https://doi.org/10.3103/S0735272723080058.