Scattering of light waves by finite metal nanostrip gratings: Nystrom-type method and resonance effects
DOI:
https://doi.org/10.3103/S0735272715050027Keywords:
thin nanostrip, generalized boundary conditions, singular integral equations, hypersingular integral equations, Nystrom-type method, scattering and absorption of waves, plasmon, grating resonanceAbstract
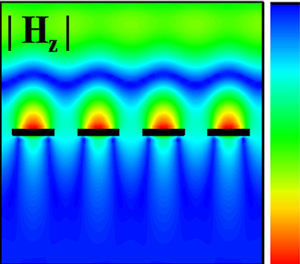
Efficient and rapidly convergent numerical algorithm for the simulation of the scattering of light waves by a finite gratings consisting of thin (thinner than the wavelength in the free space) metal nanostrips is presented. The model is based on the utilization of generalized boundary conditions (GBC), which allow one to exclude from consideration the field inside each strip and to reduce the two-dimensional boundary problem to one-dimensional systems of singular/hypersingular integral equations (IE). The obtained IE are solved numerically using the Nystrom-type method and the quadrature formulas of interpolation type, that provides guarantee convergence and controlled accuracy. The article presents the results of characteristics calculations for optical scattering and absorption by the gratings, which consist of silver nanostrips, as dependences on the width and on the thickness of the strips, and on the grating period. The nature of resonance phenomena has been investigated, namely the article presents the analysis of intensive optical scaterring and absorption in the case of excitation of plasmonic modes (plasmons) and of grating modes, which are induced by the periodicity.
References
- SØNDERGAARD, T.; BOZHEVOLNYI, S.J. Strip and gap plasmon polariton optical resonators. Phys. Stat. Sol. (B), 2008, v.245, n.1, p.9-19, DOI: http://dx.doi.org/10.1002/pssb.200743225.
- NATAROV, D.M.; BYELOBROV, V.O.; SAULEAU, R.; BENSON, T.M.; NOSICH, A.I. Periodicity-induced effects in the scattering and absorption of light by infinite and finite gratings of circular silver nanowires. Optics Express, 2011, v.19, n.22, p.22176-22190, DOI: http://dx.doi.org/10.1364/OE.19.022176.
- NATAROV, D.M.; SAULEAU, R.; NOSICH, A.I. Periodicity-enhanced plasmon resonances in the scattering of light by sparse finite gratings of circular silver nanowires. IEEE Photonics Technol. Lett., 2012, v.24, n.1, p.43-45, DOI: http://dx.doi.org/10.1109/LPT.2011.2172203.
- GHENUCHE, P.; VINCENT, G.; LAROCHE, M.; BARDOU, N.; HAÏDAR, R.; PELOUARD, J.-L.; COLLIN, S. Optical extinction in a single layer of nanorods. Phys. Rev. Lett., 2012, v.109, p.143903, DOI: http://dx.doi.org/10.1103/PhysRevLett.109.143903.
- BYELOBROV, V.O.; BENSON, T.M.; NOSICH, A.I. Binary grating of subwavelength silver and quantum wires as a photonic-plasmonic lasing platform with nanoscale elements. IEEE J. Selected Topics Quantum Electron., 2012, v.18, n.6, p.1839-1846, DOI: http://dx.doi.org/10.1109/JSTQE.2012.2213586.
- ZINENKO, T.L.; MARCINIAK, M.; NOSICH, A.I. Accurate analysis of light scattering and absorption by an infinite flat grating of thin silver nanostrips in free space using the method of analytical regularization. IEEE J. Selected Topics Quantum Electron., 2013, v.19, n.3, DOI: http://dx.doi.org/10.1109/JSTQE.2012.2227685.
- KOTTMANN, J.P.; MARTIN, O.J.F. Accurate solution of the volume integral equation for high-permittivity scatterers. IEEE Trans. Antennas Propag., Nov. 2000, v.48, n.11, p.1719-1726, DOI: http://dx.doi.org/10.1109/8.900229.
- GIANNINI, V.; SÀNCHEZ-GIL, J.A. Calculations of light scattering from isolated and interacting metallic nanowires of arbitrary cross section by means of Green’s theorem surface integral equations in parametric form. JOSA A, 2007, v.24, n.9, p.2822-2830, DOI: http://dx.doi.org/10.1364/JOSAA.24.002822.
- BLESZYNSKI, E.; BLESZYNSKI, M.; JAROSZEWICZ, T. Surface-integral equations for electromagnetic scattering from impenetrable and penetrable sheets. IEEE Antennas Propag. Magazine, Dec. 1993, v.35, n.6, p.14-25, DOI: http://dx.doi.org/10.1109/74.248480.
- SHAPOVAL, O.V.; SAULEAU, R.; NOSICH, A.I. Scattering and absorption of waves by flat material strips analyzed using generalized boundary conditions and Nystrom-type algorithm. IEEE Trans. Antennas Propag., Sept. 2011, v.59, n.9, p.3339-3346, DOI: http://dx.doi.org/10.1109/TAP.2011.2161547.
- BALABAN, M.V.; SMOTROVA, E.I.; SHAPOVAL, O.V.; BULYGIN, V.S.; NOSICH, A.I. Nystrom-type techniques for solving electromagnetics integral equations with smooth and singular kernels. Int. J. Numer. Model.: Electronic Networks, Devices and Fields, 2012, v.25, Nos. 5-6, p.490-511, DOI: http://dx.doi.org/10.1002/jnm.1827.
- JOHNSON, P.B.; CHRISTY, R.W. Optical constants of the noble metals. Phys. Rev. B, 1972, v.6, n.12, p.4370-4379, DOI: http://dx.doi.org/10.1103/PhysRevB.6.4370.
- GANDEL’, Y.V.; KONONENKO, A.S. Justification of the numerical solution of a hypersingular integral equation. Differential Equations, 2006, v.42, n.9, p.1326-1333, DOI: http://dx.doi.org/10.1134/S0012266106090114.
- SUKHAREVSKY, I.O.; SHAPOVAL, O.V.; NOSICH, A.I.; ALTINTAS, A. Validity and limitations of the median-line integral equation technique in the scattering by material strips of sub-wavelength thickness. IEEE Trans. Antennas and Propagation, July 2014, v.62, n.7, p.3623-3631, DOI: http://dx.doi.org/10.1109/TAP.2014.2316295.